12 13 As A Decimal

Representation of the fourth dimension of mean solar day using decimally related units

GMT at page generation (last updated 22 October 2022) ( update )
24-60 minutes time	Decimal fourth dimension
23:25:15	nine:75:86
	9^h 75^thou 86^{due south}
	0.97586 d

French decimal clock from the time of the French Revolution. The large dial shows the x hours of the decimal twenty-four hour period in Standard arabic numerals, while the small punch shows the ii 12-60 minutes periods of the standard 24-hour day in Roman numerals.

Decimal-time clock reading 2.50 DT equivalent to 06:00 standard fourth dimension

Decimal time is the representation of the time of 24-hour interval using units which are decimally related. This term is ofttimes used specifically to refer to the time system used in French republic for a few years beginning in 1792 during the French Revolution, which divided the day into 10 decimal hours, each decimal 60 minutes into 100 decimal minutes and each decimal infinitesimal into 100 decimal seconds ( 100000 decimal seconds per day), every bit opposed to the more familiar UTC fourth dimension standard, which divides the solar day into 24 hours, each hour into threescore minutes and each minute into 60 seconds ( 86400 SI seconds per twenty-four hour period).

The primary advantage of a decimal fourth dimension arrangement is that, since the base used to divide the time is the same as the one used to correspond it, the whole time representation can be handled equally a single cord. Therefore, information technology becomes simpler to interpret a timestamp and to perform conversions. For case, 1:23:45 is 1 decimal hour and 23 decimal minutes and 45 decimal seconds, or i.2345 decimal hours, or 123.45 decimal minutes or 12345 decimal seconds; 3 hours is 300 minutes or 30,000 seconds. This holding also makes it straightforward to represent a timestamp as a fractional day, and then that 2022-10-22.54321 tin can be interpreted every bit five decimal hours and 43 decimal minutes and 21 decimal seconds after the kickoff of that day, or a fraction of 0.54321 (54.321%) through that day (which is shortly subsequently traditional xiii:00). It also adjusts well to digital time representation using epochs, in that the internal fourth dimension representation tin exist used direct both for computation and for user-facing display.

decimal	24-hr	12-hour
0 (Midnight)	00:00	12:00 a.m.
one	02:24	two:24 a.m.
ii	04:48	4:48 a.m.
iii	07:12	vii:12 a.k.
4	09:36	9:36 a.chiliad.
v (Noon)	12:00	12:00 p.m.
vi	14:24	2:24 p.k.
seven	16:48	4:48 p.g.
8	19:12	7:12 p.m.
ix	21:36	9:36 p.m.

History [edit]

China [edit]

Decimal fourth dimension was used in China throughout most of its history alongside duodecimal fourth dimension. The midnight-to-midnight 24-hour interval was divided both into 12 double hours (traditional Chinese: 時辰; simplified Chinese: 时辰; pinyin: shí chén ) and besides into x shi / 100 ke (Chinese: 刻; pinyin: kè ) by the 1st millennium BC.^[one] ^[2] Other numbers of ke per day were used during three curt periods: 120 ke from 5 to three BC, 96 ke from 507 to 544 CE, and 108 ke from 544 to 565. Several of the roughly fifty Chinese calendars as well divided each ke into 100 fen, although others divided each ke into 60 fen. In 1280, the Shoushi (Season Granting) calendar further subdivided each fen into 100 miao, creating a complete decimal fourth dimension system of 100 ke, 100 fen and 100 miao.^[3] Chinese decimal fourth dimension ceased to be used in 1645 when the Shíxiàn calendar, based on European astronomy and brought to China by the Jesuits, adopted 96 ke per twenty-four hours alongside 12 double hours, making each ke exactly one-quarter hour.^[4]

French republic [edit]

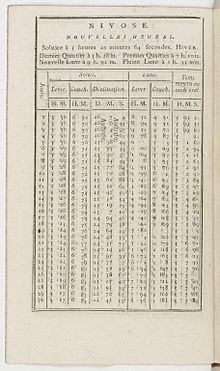
Astronomical table from the Almanach national de France using decimal fourth dimension

In 1754, Jean le Rond d'Alembert wrote in the Encyclopédie:

It would be very desirable that all divisions, for case of the livre, the sou, the toise, the day, the 60 minutes, etc. would be from tens into tens. This partition would event in much easier and more convenient calculations and would be very preferable to the arbitrary division of the livre into twenty sous, of the sou into twelve deniers, of the solar day into twenty-four hours, the hour into sixty minutes, etc.^[5] ^[half-dozen]

In 1788, Claude Boniface Collignon proposed dividing the twenty-four hours into 10 hours or 1000 minutes, each new 60 minutes into 100 minutes, each new minute into 1000 seconds, and each new 2nd into 1000 tierces (Latin for "third"). The distance the twilight zone travels in one such tierce at the equator, which would be ane-billionth of the circumference of the earth, would exist a new unit of length, provisionally chosen a half-handbreadth, equal to iv modern centimetres. Further, the new tierce would be divided into chiliad quatierces, which he called "microscopic points of time". He also suggested a week of 10 days and dividing the year into 10 "solar months".^[seven]

Decimal fourth dimension was officially introduced during the French Revolution. Jean-Charles de Borda made a proposal for decimal fourth dimension on 5 November 1792. The National Convention issued a prescript on five October 1793:

Xi. Le jour, de minuit à minuit, est divisé en dix parties, chaque partie en dix autres, ainsi de suite jusqu'à la plus petite portion commensurable de la durée.

XI. The mean solar day, from midnight to midnight, is divided into ten parts, each part into ten others, so forth until the smallest measurable portion of elapsing.

These parts were named on 24 November 1793 (iv Frimaire of the Year Ii). The primary divisions were called hours, and they added:

La centième partie de l'heure est appelée minute décimale; la centième partie de la infinitesimal est appelée seconde décimale. (accent in original)

The hundredth part of the hour is called decimal minute; the hundredth role of the minute is called decimal 2nd.

French timepiece with 12-hour (upper) and decimal (lower) faces, 1793–94

Thus, midnight was called either dix heures ("ten hours") or nada heures, noon was called cinq heures ("five hours"), etc. Units were either written out or abbreviated, such as 8 h. 72 yard. Sometimes in official records, decimal hours were divided into tenths, or décimes, instead of minutes (e.1000., 8.7 h.).^[8] ^[nine] Although clocks and watches were produced with faces showing both standard fourth dimension with numbers 1–24 and decimal fourth dimension with numbers ane–10, decimal time never defenseless on; it was not officially used until the kickoff of the Republican twelvemonth Three, 22 September 1794, and mandatory use was suspended 7 April 1795 (eighteen Germinal of the Yr Iii), in the same law which introduced the original metric system. Thus, although decimal time is sometimes referred to as metric time, the metric arrangement at showtime had no time unit, and later versions of the metric system used the second, equal to 1/86400 day, as the metric fourth dimension unit. In spite of this, decimal time was used in many cities, including Marseille and Toulouse, where a decimal clock with only an hour mitt was on the front of the Capitole for five years.^[viii] On the Palace of the Tuileries in Paris, 2 of the four clock faces displayed decimal time until at least 1801.^[x] The mathematician and astronomer Pierre-Simon Laplace had a decimal watch made for him, and used decimal time in his piece of work, in the form of fractional days.

Decimal time was part of a larger attempt at decimalisation in revolutionary French republic (which likewise included decimalisation of currency and metrication) and was introduced as part of the French Republican Calendar, which, in addition to decimally dividing the twenty-four hours, divided the month into three décades of x days each; this calendar was abolished at the end of 1805. The start of each year was determined according to the day of the autumnal equinox, in relation to true or apparent solar time at the Paris Observatory. Decimal time would also accept been reckoned according to apparent solar time, depending on the location it was observed, as was already the do mostly for the setting of clocks. 23:25:15 GMT is 9 h 82 min 36 s decimal fourth dimension in Paris.

At the International Acme Conference of 1884, the following resolution was proposed past the French delegation and passed nem con (with 3 abstentions):

VII. That the Conference expresses the hope that the technical studies designed to regulate and extend the application of the decimal system to the division of angular space and of fourth dimension shall exist resumed, so as to permit the extension of this application to all cases in which it presents real advantages.

In the 1890s, Joseph Charles François de Rey-Pailhade, president of the Toulouse Geographical Society, proposed dividing the day into 100 parts, called cés, equal to 14.4 standard minutes, and each divided into 10 decicés, 100 centicés, etc. The Toulouse Sleeping accommodation of Commerce adopted a resolution supporting his proposal in April 1897. Although widely published, the proposal received little backing.^[eleven]

The French made another attempt at the decimalization of time in 1897, when the Commission de décimalisation du temps was created by the Bureau des Longitudes, with the mathematician Henri Poincaré as secretary. The committee adopted a compromise, originally proposed by Henri de Sarrauton of the Oran Geographical Society, of retaining the 24-hour twenty-four hour period, but dividing each hour into 100 decimal minutes, and each minute into 100 seconds. The programme did not gain acceptance and was abandoned in 1900.

Switzerland [edit]

On 23 October 1998, the Swiss scout company Swatch introduced a decimal time called Internet Fourth dimension, which divides the day into 1,000 decimal minutes (Swatch called them .beats), (each 86.iv seconds in standard time) counted from 000–999, with @000 existence midnight and @500 being noon standard time in Switzerland, which is Central European Time (1 hour alee of Universal Time). A line painted on Swatch headquarters in the Swiss city of Biel (at present Biel/Bienne) was declared to mark the Biel Meridian, and Primal European Fourth dimension was relabelled as "Biel Meantime", even though it does not correspond to local hateful time in Biel.^[12]

Conversions [edit]

At that place are exactly 86,400 standard seconds (see SI for the current definition of the standard second) in a standard day, but in the French decimal fourth dimension system there were 100,000 decimal seconds in the day; thus, the decimal second was thirteen.6% shorter than its standard analogue.

Unit	Seconds (SI)	Minutes	Hours	h:mm:ss.sss
ane Decimal second	0.864	0.0144	0.00024	0:00:00.864
ane Decimal minute	86.4	1.44	0.024	0:01:26.400
i Decimal hour	eight,640	144	two.4	2:24:00.000

Decimal hours [edit]

Another common type of decimal fourth dimension is decimal hours. In 1896, Henri de Sarrauton of the Oran Geographical Society proposed dividing the 24 hours of the mean solar day each into 100 decimal minutes, and each minute into 100 decimal seconds.^[13] Although endorsed by the Agency des Longitudes, this proposal failed, but using decimal fractions of an 60 minutes to represent the time of twenty-four hours instead of minutes has get common.

Decimal hours are oftentimes used in accounting for payrolls and hourly billing. Time clocks typically record the time of solar day in tenths or hundredths of an hour. For case, 08:xxx would exist recorded equally 08.50. This is intended to make accounting easier past eliminating the need to convert betwixt minutes and hours.

For aviation purposes, where it is common to add times in an already complicated environment, time tracking is simplified by recording decimal fractions of hours. For instance, instead of calculation 1:36 to ii:36, getting 3:72 and converting it to 4:12, one would add 1.6 to 2.vi and get iv.ii hours.^[14]

Partial days [edit]

The time of solar day is sometimes represented as a decimal fraction of a day in scientific discipline and computers. Standard 24-hour time is converted into a fractional day by dividing the number of hours elapsed since midnight by 24 to make a decimal fraction. Thus, midnight is 0.0 solar day, noon is 0.5 d, etc., which can be added to whatever type of date, including (all of which refer to the same moment):

Gregorian dates: 2000 January i.5
Ii-line elements: 00001.50000000
Julian dates: 2451545.0
Excel serial dates: 36526.5

Equally many decimal places may be used as required for precision, so 0.5 d = 0.500000 d. Partial days are often calculated in UTC or TT, although Julian Dates use pre-1925 astronomical date/fourth dimension (each date began at apex = ".0") and Microsoft Excel uses the local time zone of the computer. Using partial days reduces the number of units in fourth dimension calculations from iv (days, hours, minutes, seconds) to just one (days).

Fractional days are often used by astronomers to record observations, and were expressed in relation to Paris Mean Time past the 18th century French mathematician and astronomer Pierre-Simon Laplace, as in these examples:^[15]

... et la distance périhélie, égale à 1,053095; ce qui a donné cascade fifty'instant du passage au périhélie, sept.29^j,10239, temps moyen compté de minuit à Paris.

Les valeurs précédentes de a, b, h, fifty, relatives à trois observations, ont donné la distance périhélie égale à 1,053650; et pour l'instant du passage, sept.29^j,04587; ce qui diffère peu des résultats fondés sur cinq observations.

—Pierre-Simon Laplace, Traité de Mécanique Céleste

Fractional days have been used past astronomers ever since. For instance, the 19th century British astronomer John Herschel gave these examples:^[xvi]

Between Greenwich noon of the 22d and 23d of March, 1829, the 1828th equinoctial year terminates, and the 1829th commences. This happens at 0^d·286003, or at vi^h 51^thou 50^s·66 Greenwich Mean Fourth dimension ... For example, at 12^h 0^m 0^{due south} Greenwich Mean Time, or 0^d·500000...

—John Herschel, Outlines of Astronomy

Fractional days are commonly used to express epochs of orbital elements. The decimal fraction is usually added to the calendar date or Julian day for natural objects, or to the ordinal date for artificial satellites in ii-line elements.

Decimal multiples and fractions of the second [edit]

The second is the International Organization of Units (SI) unit of time duration. Information technology is also the standard single-unit fourth dimension representation in many programming languages, about notably C, and function of UNIX/POSIX standards used by Linux, Mac Bone X, etc.; to convert fractional days to fractional seconds, multiply the number by 86400. Partial seconds are represented as milliseconds (ms), microseconds (μs) or nanoseconds (ns). Accented times are ordinarily represented relative to 1 January 1970, at midnight UT. Other systems may use a different cipher point (like Unix time).

In principle, time spans greater than one second may be given in units such as kiloseconds (ks), megaseconds (Ms), gigaseconds (Gs), and and then on. Occasionally, these units can be found in technical literature, but traditional units like minutes, hours, days and years are much more common, and are accustomed for apply with SI.

It is possible to specify the time of day as the number of kiloseconds of elapsed fourth dimension since midnight. Thus, instead of maxim 3:45 p.m. 1 could say (time of twenty-four hours) 56.vii ks. In that location are exactly 86.4 ks in one solar day. Still, this nomenclature is rarely used in do.

Scientific decimal time [edit]

Scientists often record time equally decimal. For example, decimal days separate the day into 10 equal parts, and decimal years divide the twelvemonth into ten equal parts. Decimals are easier to plot than both (a) minutes and seconds, which uses the sexagesimal numbering system, (b) hours, months and days, which has irregular calendar month lengths. In astronomy, the so-chosen Julian mean solar day uses decimal days centered on Greenwich noon.

Seconds in a decimal minute

Since there are sixty seconds in a minute, a tenth part represents 60 / 10 = vi seconds.

Conversion betwixt decimal minutes and seconds
Decimal minutes	0.one	0.ii	0.iii	0.4	0.5	0.6	0.7	0.8	0.ix	ane.0
Second	6^{due south}	12^south	18^s	24^s	30^s	36^s	42^s	48^s	54^s	60^s

Minutes in a decimal hour

Since there are 60 minutes in an hr, a tenth role represents threescore / 10 = 6 minutes.

Conversion between decimal hours and minutes
Decimal hours	0.i	0.2	0.3	0.four	0.v	0.six	0.7	0.viii	0.ix	1.0
Minutes	6^m	12^chiliad	xviii^grand	24^yard	xxx^m	36^grand	42^thousand	48^m	54^thou	60^m

Hours in a decimal day

Since there are 24 hours in a day, a tenth part represents 24 / 10 = 2.4 hours (2 hours and 24 minutes).

Conversion between decimal 24-hour interval and hours/minutes
Decimal days	0.1	0.2	0.iii	0.iv	0.5	0.half-dozen	0.7	0.viii	0.ix	1.0
Hours/minutes	2^h 24^m	four^h 48^m	7^h 12^g	9^h 36^g	12^h	14^h 24^m	16^h 48^chiliad	19^h 12^m	21^h 36¹⁰⁰⁰	24^h

Length of a decimal year

Since there are nigh 365 days in a yr, there are about 365 / 10 = 36.5 days in a tenth of a year. Hence the yr 2020.v represents the day 2 July 2020.^[17] More than exactly, a "Julian year" is exactly 365.25 days long, so a 10th of the year is 36.525 days (36 days, 12 hours, 36 minutes).

Conversion between decimal years and date (in a common year)
Decimal years	0.0	0.1	0.2	0.3	0.4	0.5	0.6	0.7	0.eight	0.ix	1.0
Days	0	36.525	73.050	109.575	146.100	182.625	219.150	255.675	292.200	328.725	365.250
Appointment Fourth dimension	1 Jan 00:00	6 February 12:36	15 Mar 01:12	20 Apr 13:48	27 May 2:24	1 Jul 15:00	8 Aug 03:36	xiii Sep 16:12	twenty October 04:48	25 November 17:24	1 Jan 06:00

These values, based on the Julian year, are most likely to be those used in astronomy and related sciences. A Gregorian year, which takes into account the 100 vs. 400 spring twelvemonth exception rule of the Gregorian calendar, is 365.2425 days (the boilerplate length of a year over a 400 year bicycle), resulting in 0.ane years existence a period of 36.52425 days ( three155 695.2 seconds; 36 days, 12 hours, 34 minutes, 55.2 seconds).

Other decimal times [edit]

Numerous individuals have proposed variations of decimal time, dividing the day into different numbers of units and subunits with different names. Most are based upon fractional days, so that one decimal fourth dimension format may exist easily converted into some other, such that all the following are equivalent:

0.500 day
5:00 French Republican Calendar
@500.beats Swatch Internet Time (see in a higher place)
fifty.0 kes or cés (centidays)
500 millidays
50.0% time as a percentage of the solar day
12:00 standard time

Some decimal fourth dimension proposals are based upon alternate units of metric time. The difference between metric time and decimal time is that metric time defines units for measuring time interval, equally measured with a stopwatch, and decimal time defines the time of 24-hour interval, every bit measured by a clock. Simply as standard time uses the metric time unit of the 2nd as its basis, proposed decimal time scales may use alternative metric units.

Epoch Days [edit]

One of such approaches is to employ epoch time represented in number of days ("decimal time as epoch day",^[18] ED) and and then grouping the digits, resulting in a decimal representation, for example, 2028-09-20 11:25:45 UTC is equal to 1853061945 UNIX seconds, thus dividing them past 86400 we get 21447.476215277777 ED (Epoch Day). Grouping the digits of epoch twenty-four hour period like so — (21, 4, 4, 7, 4, 76, 21.5277777) — results in a usable decimal date and time, where thousands are used as years (21 decimal years), hundreds as months (4 decimal months), tens as weeks (4 decimal weeks), remaining digit as days (7 days), and fractions of day similar 0.1 as hours (iv decimal hours), 0.001 equally minutes (76 decimal minutes), 0.00001 equally seconds (21.527777 decimal seconds), allowing to care for entire date as a decimal number with decimal places named past calendrical terms.

See as well [edit]

12-60 minutes clock
24-hr clock
Centesimal minute and 2d of arc
Decimal agenda
Hexadecimal time
List of unusual units of measurement
Metric fourth dimension
Stardate
Unix time

References [edit]

Notes [edit]

^ Nachum Dershowitz, Edward K. Reingold, "Calendrical Calculations", page 207
^ Joseph Needham, Ling Wang, and Derek John de Solla Cost Heavenly clockwork: the groovy astronomical clocks of medieval China (Cambridge: Cambridge Academy Press, 1986) 199-202, ISBN 0-521-32276-half-dozen.
^ Jean-Claude Martzloff, "Chinese mathematical astronomy", in Helaine Selin, ed., Mathematics across cultures (Dordrecht: Kluwer, 2000) 373–407, p. 393, ISBN 0-7923-6481-3.
^ K. Yabuuti [Kiyoshi Yabuuchi], "Astronomical tables in Mainland china, from the Wutai to the Ch'ing dynasties", in Japanese Studies in the History of Science no. 2 (1963) 94–100.
^ Vera, Hector (2009). "Decimal Time: Misadventures of a Revolutionary Idea, 1793–2008". KronoScope. Brill. ix (1–2): 31–32. doi:ten.1163/156771509X12638154745382. ISSN 1567-715X. ^{[ permanent dead link ]}
^ d'Alembert, Jean le Rond (1754). Encyclopédie. Archived from the original on 2012-12-15.
^ Collignon, Claude Boniface (1788). Découverte d'étalons justes, naturels, invariables et universels. Chez l'auteur. pp. 39–40.
^ ^a ^b Matthew Shaw (2011). Time and the French Revolution: The Republican Calendar, 1789-year XIV. Boydell & Brewer Ltd. pp. 132–3. ISBN978-0-86193-311-2.
^ Carrigan, Richard A. (May–June 1978). "Decimal Fourth dimension: Dissimilar the metric system of measurements, decimal time did not survive the French Revolution. But is dividing the day by tens a possibility for the future?". American Scientist. 66 (3): 305–313. JSTOR 27848641.
^ Ernest Leroux, ed. (1900). Bulletin de géographie historique et descriptive, année 1899. Paris: Comité des travaux historiques et scientifiques. p. 142.
^ Bulletin of the International Railway Congress (English language ed.). 1899. p. 784.
^ "Internet Time". www.swatch.com. Swatch United States.
^ Sarrauton, Henri de (1896). L'Heure décimale et la division de la circonférence, Oran: Fouque
^ "Pilot Log Books". Civil Aviation Safety Authority. Archived from the original on 2012-03-21. Retrieved 2012-06-23 .
^ Traité de Mécanique Céleste. 1823.
^ Outlines of Astronomy.
^ Campbell, Wallace Hall (2003). Introduction to geomagnetic fields (2 ed.). Cambridge University Press. p. 316. ISBN0-521-52953-0. ISBN 978-0-521-52953-2
^ "Extended Decimal Fourth dimension -- Python library". github.com/mindey/edtime. GitHub. 2021-12-twenty. Retrieved 2021-12-20 .

Sources [edit]

National Convention of the French Democracy (1793) LE CALENDRIER RÉPUBLICAIN Textes officiels Décrets Relatifs à l'établissement de 50'Ère Républicaine Archived 2013-03-17 at the Wayback Auto published by Philippe Chapelin 2002
Sizes, Inc. (2000) decimal time units Last revised 27 Feb 2004
Herschel, John (1849) Outlines of Astronomy published by Gallica 1995

External links [edit]

Decimal Fourth dimension by Kevin Gut - Website that shows the current decimal time and a converter to and from decimal time
Metric Clock past Paul Dunning / Shows the current local decimal time
Decimal Time Clock & Agenda

12 13 As A Decimal,

Source: https://en.wikipedia.org/wiki/Decimal_time

Posted by: mossstrater.blogspot.com